Basic Filter Circuits Explained
- Posted by doEEEt Media Group
- On August 17, 2022
- 0
This article published by Knowles Precision Devices Blog explains basic filter circuit background information on how filters do what they do.
Regardless of the technology behind the filter, there are several key concepts that all filters share that we will dive into throughout this series.
At the most basic level, filters are necessary for RF devices so that unwanted frequencies do not pass through the circuit and cause interference. While filtering can become quite complex as operating frequencies increase, it can be made much less daunting by having an understanding of how basic filters are built using standard electrical components such as resistors (Rs), capacitors (Cs), and inductors (Ls).
Before we jump into types of filters, though, let’s start by looking at how a voltage divider functions. A voltage divider is a passive linear circuit that produces an output voltage (Vo) fraction of the input voltage (Vi). As shown in Figure 1, the voltage divider changes the Vi down to the Vo based on the resistors’ values, which are R1 and R2 below.
If we start with Ohms Law, which as a reminder, states that electric current is proportional to voltage and inversely proportional to resistance since resistance hinders the flow of current in the circuit, we can derive the following formula for how a voltage divider works:
![voltage divider equation [1]](https://www.doeeet.com/content/wp-content/uploads/2022/08/voltage-divider-equation-1.png)
voltage divider equation [1]
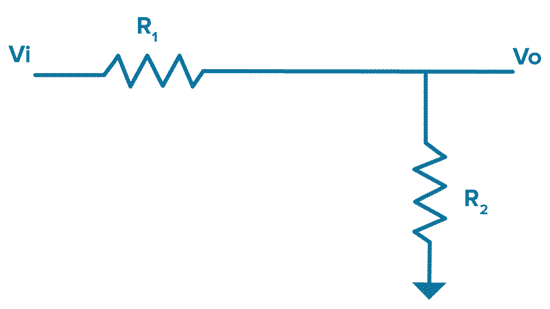
Figure 1 illustrates the very familiar DC circuit, the voltage divider.
As you can see from the formula above, as R1 grows (or as R2 shrinks), the output voltage will drop.
Swapping Rs and Cs to Turn Our Voltage Divider into a Filter
Now that we know how our simple voltage divider works let’s look at what happens if we trade out some Rs for Cs. The impedance of a capacitor will change with frequency, and that capacitance can be calculated as follows:
![voltage divider equation [1]](https://www.doeeet.com/content/wp-content/uploads/2022/08/capacitance-capacitive-impedance-equation-2.png)
voltage divider equation [1]
- Z = Impedance
- Omega = Angular Frequency
- i is the imaginary number since we are using complex numbers here
- C = Capacitance
Thus, for a capacitor, impedance decreases with frequency. So, if we swap R2 for a C, as shown in Figure 2, we will have a low-pass RC filter, a filter circuit that passes frequency signals below a certain cutoff frequency and blocks frequency signals higher than that point.
RC Low Pass Filter
In the RC low-pass filter, the path to the ground goes through a capacitor, which means impedance will decrease with increasing frequency. Therefore, in this circuit, the ratio of the Vi down to the Vo will depend on the values of R and C and the signal’s frequency. With high frequencies, impedance is low, and energy is sent to the ground as Vi is divided. Low frequencies see a higher impedance, sending energy to the output.

Figure 2. A diagram of an RC low-pass filter.

Figure 3. Output voltage decreases with increasing frequency in an RC low-pass filter.
If we do this the other way around and replace R1 with a C, the path to output goes through the capacitor, and we get the opposite effect.
RC High Pass Filter
In this configuration, low frequencies see impedance higher than R, so the low-frequency signals go to the ground while the high-frequency signals see an impedance lower than R, which means we get a high-frequency output.
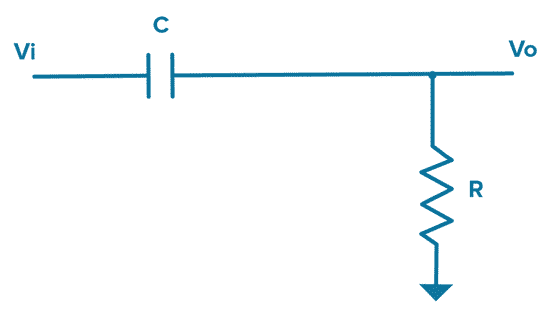
Figure 4. A diagram of an RC high-pass filter.
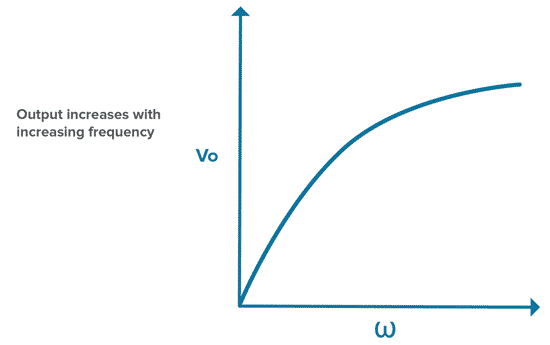
Figure 5. Output voltage increases with increasing frequency when using a high-pass RC filter.
Swapping Cs and Ls to Create Your Desired Filter
Inductors have the opposite response to frequency than capacitors. This can be calculated as follows:
This means that impedance increases with frequency in an inductor. To create a low-pass filter using an R and L, we can start with an RC high-pass filter, as shown in Figure 4, and swap the C for an L. We can also take the same approach to designing a high-pass filter if we start with an RC low-pass filter, as shown in Figure 2, and replace the C with an L. Both examples are illustrated in Figure 6.

Figure 6. The top diagram converts a low-pass filter to a high-pass filter by swapping the C for an L in the path to the ground. The bottom diagram converts the high-pass filter to a low-pass filter by swapping the C for an L in the output line.
As it turns out, RC filters don’t provide the best performance in terms of roll-off, which is the slope over frequency from passing a signal to blocking it. To improve this, RF designers can combine Cs and Ls, balancing their opposite responses to frequency to build different low-pass, high-pass, and band-pass LC filter responses, as shown in Figure 7.
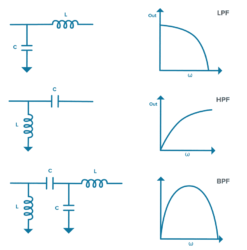
Figure 7. Different types of LC filters and their frequency responses.
Manipulating Impedance to Achieve the Desired Frequency Response in Your Filter
Given that Rs, Cs, and Ls offer different variations on impedance, we can think of designing a filter response as manipulating different impedances to achieve the desired frequency response. Thinking back to the DC voltage divider we started with at the beginning of this post, we can build on this to create a high pass circuit by adding networks of impedance (Zs) using various combinations of RCs and LCs, as discussed throughout this post.

Figure 8. This example replaces L and C with impedances Z1 and Z2.
Figure 8 below shows a high pass circuit where Z1 decreases with frequency and Z2 increases with frequency. In general, we can create more complex high-performance filters by replacing individual Rs, Cs, and Ls with sections of a circuit with very specific impedance vs. frequency behavior.
By breaking down the different ways to use the frequency dependencies of Cs and Ls, you can see how it is possible to get a variety of different filter responses and form simple filters that can serve as the building blocks for more complex filtering needs.
Five key filter specifications to understand and check can be followed in the related articles here.
- Space-Grade components available for immediate delivery - April 10, 2025
- Exclusive stock on doEEEt: How to access and request - April 10, 2025
- Managing EEE components for LEO and lower cost space missions - December 17, 2024


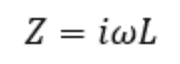
0 comments on Basic Filter Circuits Explained